Забезпечення теплового режиму роботи стержневих конструкцій п’єзокерамічних електроакустичних перетворювачів
Основний зміст сторінки статті
Анотація
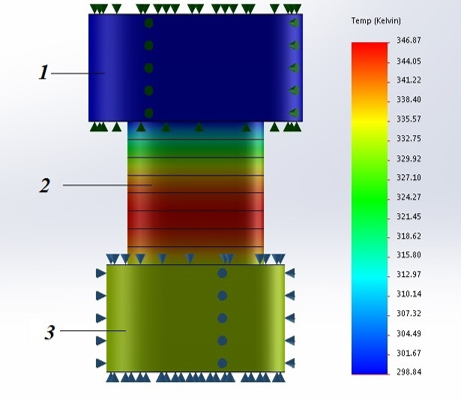
Розглянуто два методи аналізу теплових полів для конструкцій п'єзокерамічних електроакустичних перетворювачів – аналітичний розрахунок та моделювання. Проаналізовано причини виникнення нагріву, а також негативні наслідки, до яких призводить нагрів перетворювачів. Обґрунтовано необхідність аналізу теплових полів саме для п'єзокерамічних електроакустичних перетворювачів стержневої конструкції. Проведено порівняння аналітичного методу знаходження теплового поля, заснованого на розв'язанні диференціального рівняння теплопровідності Фур'є, і комп'ютерного моделювання, виконаного за допомогою методу скінченних елементів в системі автоматизованого проектування SolidWorks. Проведено чисельні розрахунки теплових полів розглянутими методами для типової конструкції стержневого перетворювача. Показано процес розігріву електроакустичного перетворювача. Встановлено, що моделювання дозволяє врахувати конструкційні особливості перетворювачів і дозволяє швидше змінювати параметри елементів для пошуку найбільш раціонального конструкторського рішення. Отримані результати можуть бути використані при конструюванні стержневих п'єзокерамічних електроакустичних перетворювачів.
Блок інформації про статтю

Ця робота ліцензується відповідно до Creative Commons Attribution 4.0 International License.
Автори, які публікуються у цьому журналі, погоджуються з наступними умовами:- Автори залишають за собою право на авторство своєї роботи та передають журналу право першої публікації цієї роботи на умовах ліцензії Creative Commons Attribution License, котра дозволяє іншим особам вільно розповсюджувати опубліковану роботу з обов'язковим посиланням на авторів оригінальної роботи та першу публікацію роботи у цьому журналі.
- Автори мають право укладати самостійні додаткові угоди щодо неексклюзивного розповсюдження роботи у тому вигляді, в якому вона була опублікована цим журналом (наприклад, розміщувати роботу в електронному сховищі установи або публікувати у складі монографії), за умови збереження посилання на першу публікацію роботи у цьому журналі.
- Політика журналу дозволяє і заохочує розміщення авторами в мережі Інтернет (наприклад, у сховищах установ або на особистих веб-сайтах) рукопису роботи, як до подання цього рукопису до редакції, так і під час його редакційного опрацювання, оскільки це сприяє виникненню продуктивної наукової дискусії та позитивно позначається на оперативності та динаміці цитування опублікованої роботи (див. The Effect of Open Access).
Посилання
V. S. Didkovskiy, S. M. Poroshin, O. G. Leyko, А. О. Leyko, and O. I. Drozdenko, Construction of electroacoustic devices and systems for multimedia acoustic technology. K.: NTUU “KPI,” 2013.
G. Hmelev, V. Barsukov, and E. Ilchenko, “Study of the effect of temperature on the parameters of ultrasonic oscillatory systems,” Polzunovsky Alm., vol. 1, pp. 54–58, 2013.
O. Kalisz et al., “Thermographic evaluation of healing process on patients after surgery of cataract with the use of facoemulsyphication,” in Proceedings of the 2008 International Conference on Quantitative InfraRed Thermography, 2008, DOI: 10.21611/qirt.2008.03_04_17.
S. I. Kosuba and S. V. Skicuk, “The effectiveness of pre-cooling the irrigation solution for cataract phacoemulsification,” Ophthalmol. J., vol. 5, pp. 27–28, 2008.
B. M. Aznabaev, Ultrasonic cataract surgery - phacoemulsification, August Bor. Moscow, 2005, ISBN: 5-901053-21-4.
A. C. H. Tan and F. S. Hover, “On the influence of transducer heating in underwater ultrasonic thrusters,” 20th International Congress on Acoustics (ICA 2010), 2010, no. August, pp. 371–377. URL: https://www.acoustics.asn.au/conference_proceedings/ICA2010/cdrom-ICA2010/papers/p368.pdf.
M.V. Bogush, E.M. Bogush, and E.M. Pikalev, “Analysis of temperature stresses in the elements of hydroacoustic antennas,” Instrumentation, vol. 10, no. 160, pp. 38–42, 2013. ISSN: 2071-7865
O. Drozdenko, K. Drozdenko, and L. Perchevska, “Features of thermal fields calculation for cylindrical piezoceramic transducers with compensated design,” in 2018 IEEE Ukraine Student, Young Professional and Women in Engineering Congress (UKRSYW), 2018, pp. 44–47.
F. Huang, D.-Y. Zheng, S.-M. Hu, and G.-G. Peng, “The Influence of Environment Temperature on the Degradation of Lead Zirconate Titanate Ceramic”, International Conference on Material Science and Applications (Icmsa 2015), 2015, vol. 3, pp. 723–727. DOI: 10.2991/icmsa-15.2015.132
F. Baptista, D. Budoya, V. Almeida, and J. Ulson, “An Experimental Study on the Effect of Temperature on Piezoelectric Sensors for Impedance-Based Structural Health Monitoring,” Sensors, vol. 14, no. 1, pp. 1208–1227, Jan. 2014, DOI: 10.3390/s140101208.
K. S. Drozdenko, “Single-channel focusable acoustothermometer for measuring the internal temperature of biological object,” Radioelectron. Commun. Syst., vol. 56, no. 4, pp. 207–211, Apr. 2013, DOI: 10.3103/S0735272713040067.
J. Ilg, S. J. Rupitsch, and R. Lerch, “Impedance-Based Temperature Sensing With Piezoceramic Devices,” IEEE Sens. J., vol. 13, no. 6, pp. 2442–2449, Jun. 2013, DOI: 10.1109/JSEN.2013.2256121.
Y. Su, Y. Liu, D. Vasic, F. Costa, W. Wu, and K. Lee, “Power Enhancement of Piezoelectric Transformer by Adding Thermal Dissipation Layers,” in ICAST2013: 24th International Conference on Adaptive Structures and Technologies, 2013, pp. 239–250.
H. Li, Z. D. Deng, and T. J. Carlson, “Piezoelectric Materials Used in Underwater Acoustic Transducers,” Sens. Lett., vol. 10, no. 3, pp. 679–697, Mar. 2012, DOI: 10.1166/sl.2012.2597.
X. Lu, J. Hu, H. Peng, and Y. Wang, “A new topological structure for the Langevin-type ultrasonic transducer,” Ultrasonics, vol. 75, pp. 1–8, 2017, DOI: 10.1016/j.ultras.2016.11.008.
V. T. Rathod, “A Review of Electric Impedance Matching Techniques for Piezoelectric Sensors, Actuators and Transducers,” Electronics, vol. 8, no. 2, p. 169, Feb. 2019, DOI: 10.3390/electronics8020169.
M. R. Karafi and F. Khorasani, “Evaluation of mechanical and electric power losses in a typical piezoelectric ultrasonic transducer,” Sensors Actuators, A Phys., vol. 288, no. 2019, pp. 156–164, 2019, DOI: 10.1016/j.sna.2018.12.044.
L.K. Sienkiewicz, “Concept, implementation and analysis of the piezoelectric resonant sensor/actuator for measuring the aging process of human skin”, INP Toulouse, 2016. https://oatao.univ-toulouse.fr/16248/1/Sienkiewicz_Lukasz.pdf.
A. M. Al-Jumaily and A. Meshkinzar, “On the Development of Focused Ultrasound Liquid Atomizers,” Adv. Acoust. Vib., vol. 2017, pp. 1–10, 2017, DOI: 10.1155/2017/7861726.
A. Lozano, H. Amaveda, F. Barreras, X. Jorda`, and M. Lozano, “High-Frequency Ultrasonic Atomization With Pulsed Excitation,” J. Fluids Eng., vol. 125, no. 6, pp. 941–945, Nov. 2003, DOI: 10.1115/1.1603301.